SSC CPO QUANT REVISION BOOSTER QUIZ-4

Attempt now to get your rank among 24 students!

Question 1:

If the number $A 9257 B 684$ is divisible by 11 , then what is the least value of $A-B$ ?

Question 2:

The compound interest (in Rs., to the nearest integer) on Rs. 8,950 for 2 years at the rate of $9 \%$ per annum, compounded annually, is:

Question 3:

What is the value of $\sin ^{2} 60+\tan ^{2} 45+\sec ^{2} 45-\operatorname{cosec}^{2} 30 ?$

Question 4:

The sum of the radius of the base and the height of a closed solid cylinder is $12.5 \mathrm{~cm}$. If the total surface area of the cylinder is $275 \mathrm{~cm}{ }^{2}$, then its radius is: (Take $\left.\pi=\frac{22}{7}\right)$

Question 5:

In $\triangle \mathrm{ABC}$, if $\angle \mathrm{B}=90^{\circ}, \mathrm{AB}=21 \mathrm{~cm}$ and $\mathrm{BC}=20 \mathrm{~cm}$, then $\frac{1+\sin \mathrm{A}-\cos \mathrm{A}}{1+\sin \mathrm{A}+\cos \mathrm{A}}$ is equal to:

Question 6:

If $x^{4}+\frac{1}{x^{4}}=6887$, then the positive value of $x-\frac{1}{x}$ is:

Question 7:

The pie chart shows the money spent by Aditya through credit cards of different banks. The total money spent by him through the credit cards in a year is Rs. 360,000

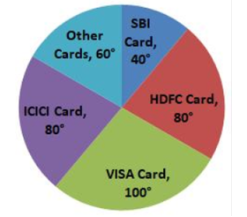
What is the maximum amount spent by Aditya through the credit card of one bank?

Question 8:

The three medians RQ, SP and TN of $\triangle \mathrm{RST}$ intersect at point $\mathrm{O}$. If the area of $\triangle \mathrm{RST}$ is $48 \mathrm{~cm}^{2}$, then the area of the quadrilateral SQON is:

Question 9:

If $8 a^{3}+b^{3}=16$ and $2 a+b=4$, then find the value of $16 a^{4}+b^{4}$

Question 10:

In $\triangle \mathrm{ABC}$, medians $\mathrm{BE}$ and $\mathrm{CF}$ are perpendicular to each other and intersect at $\mathrm{M}$. If $\mathrm{BE}=9 \mathrm{~cm}$ and $\mathrm{CF}=13 \mathrm{~cm}$, then the area of $\triangle \mathrm{ABC}$ will be:

serach-testwale
x