Question 7:
Comprehension:-
Read the information carefully and answer the question given below it :
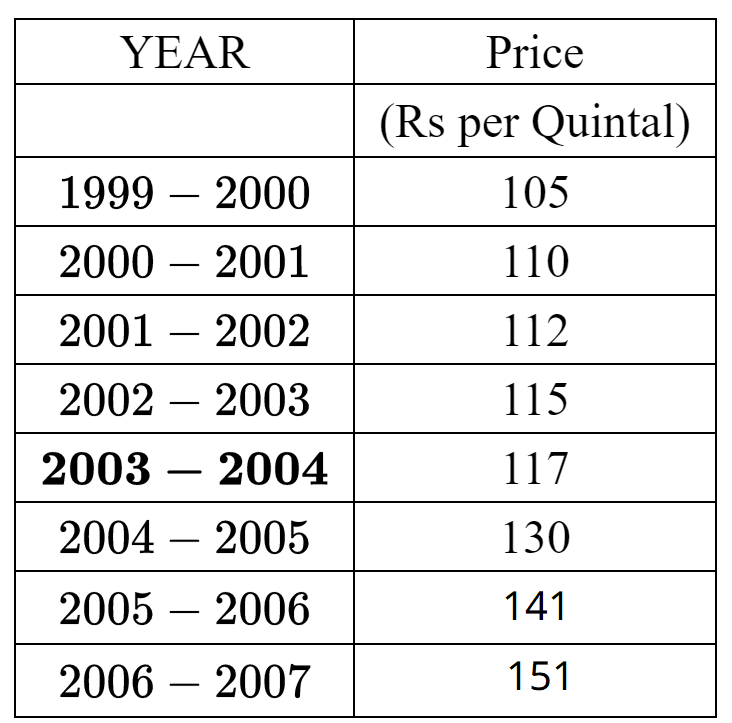
Raw cotton Procurement of a Spinning Mill.
What is the average yearly increase in raw cotton procurement prices for the spinning Mill from 1999 to 2007?
धारणा:-
निम्नलिखित जानकारी को ध्यान से पढ़िये और नीचे दिए गए प्रश्नों के उत्तर दीजिये:
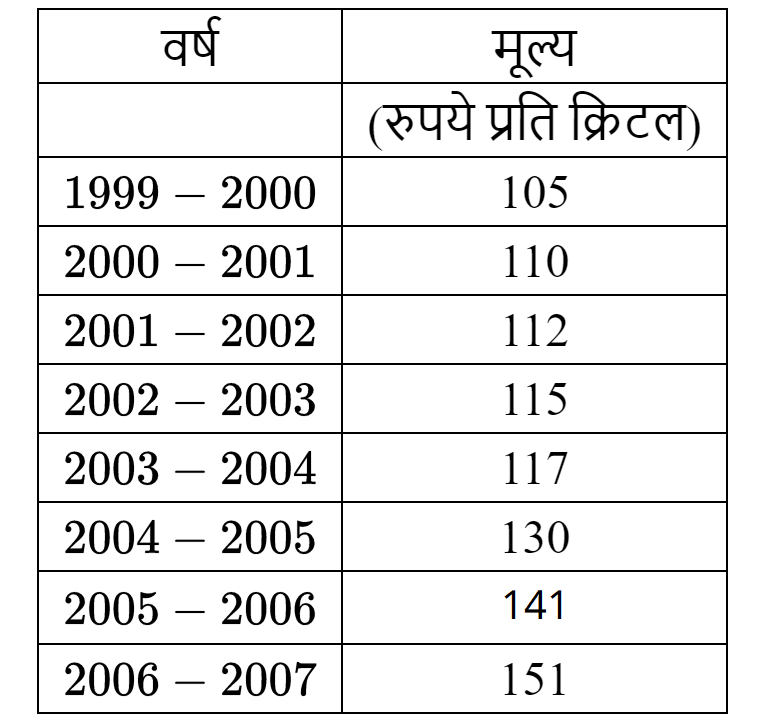
कताई मिल के कच्चे कपास की खरीद के मूल्य।
कताई मिल के लिए कच्चे कपास की खरीद के मूल्यों में 1999 से 2007 तक की औसत वार्षिक वृद्धि क्या है?
Question 8:
Jyoti invests Rs.50,000 at $5 \%$ per annum compound interest for two years. The amount standing to her credit at the end of the second year is:
ज्योति 5% वार्षिक चक्रवृद्धि ब्याज पर दो वर्षों के लिए 50,000 रुपये का निवेश करती है। दूसरे वर्ष के अंत में उसके खाते में जमा राशि है:

